Noting the Novel

When the Common Core State Standards for Mathematics (CCSS-M) were first released, one of the aspects of the reactions that I found most interesting was the diversity of reasons for opposing them. Education researchers and developers, like myself, were dismayed at the nearly exclusive focus on arithmetic in elementary school, or the undue burden placed on the middle school grade band when content was both moved up from elementary school and down from high school, or likely some other issues related to content distribution. Teachers shared some of these content concerns and also worried the standards would only place greater emphasis on standardized tests. Conservative politicians disliked the standards because they were a strong step in the direction of federal control of education. Most of the criticism I heard from the general public, though, related to the idea that the standards made mathematics unduly complicated or even incomprehensible.

This video is one example of the kinds of critiques that floated around the Internet. It’s not very long, so if you can, take a minute or two to watch it. In a nutshell, it compares the steps for the subtraction algorithm most adults learned in school to the steps of a different approach, when applied to a particular problem. The new approach — a method that I and many of my math education colleagues call counting up — comes off looking complicated and nonsensical in comparison to the traditional method.

A friend of mine posted a link to the video on my Facebook wall and asked my opinion. Here was my response. (Spolier alert: I found videos like this super annoying at the time.)

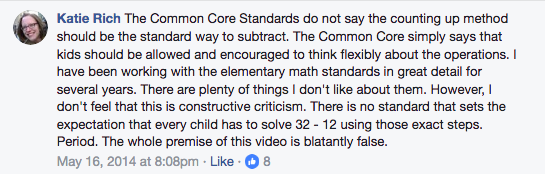
I was annoyed, first of all, that the video made it sound like this method was the new standard, which isn’t true. The standards still require students to add and subtract with the same traditional algorithm as always by the end of fourth grade. It’s just that before that, there are standards that specify that students should add and subtract (and later, multiply and divide) using more generalized strategies that help illuminate conceptual understanding of the operations. For example, consider this first-grade standard:

Add within 100, including adding a two-digit number and a one-digit number, and adding a two-digit number and a multiple of 10, using concrete models or drawings and strategies based on place value, properties of operations, and/or the relationship between addition and subtraction; relate the strategy to a written method and explain the reasoning used. Understand that in adding two-digit numbers, one adds tens and tens, ones and ones; and sometimes it is necessary to compose a ten. (CCSSI, 2010, p. 16)

That’s as specific as the standards get when it comes to prescribing computation strategies (until they require the standard, familiar algorithms later). So the video is based on a false premise. That’s the first problem.

Later, a comment from a friend on the same Facebook thread led me to respond to the second, and probably more important problem I noted at the time: the fact that the method presented in the video is based on kinds of everyday reasoning that make perfect sense in some situations.

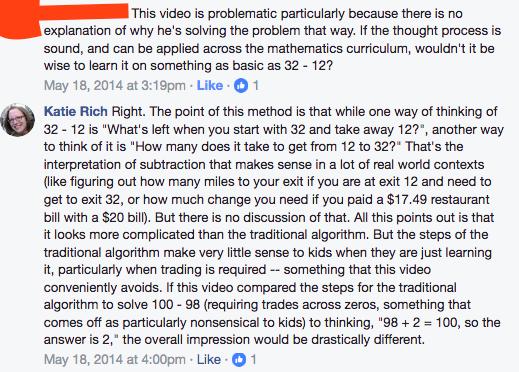
So, the second thing that really bothered me was the way that the counting-up method was (likely deliberately) set up to look confusing, when the same kind of argument could easily be made in the other direction. I felt that videos like this were masking the value of alternative strategies when I have, in my own personal and professional experiences, seen such strategies really help students see mathematics as something other than a set of procedures to be memorized and applied.

Those were my arguments pushing back on criticism of alternative computation methods at the time. I still agree with them, but also admit that they don’t always change people’s minds. My kid doesn’t like this stuff, they tell me. You’re saying it’s better and equally clear, but it’s not working for my kid, and what’s worse is that I can’t help with homework. It makes both of us feel helpless.

I’m sympathetic to these concerns, and haven’t had a better response in the past than, Hang in there. I knew it was true that these alternative methods and ideas were not magically going to make every kid suddenly understand computation in a new way. Education is not so simple. I was claiming that methods like counting-up subtraction often made sense — but what about the cases when it doesn’t?

I read a few things in the past few months that got me thinking about this. First, I read the first three chapters of Jerome Bruner’s Acts of Meaning. In his third chapter, as he constructs an explanation for patterns of language acquisition in young children, Bruner (1990) points out that from infancy, children have a strong tendency to devote more attention to things that are novel:

[W]hen [children] begin acquiring language they are much more likely to devote their linguistic efforts to what is unusual in their world. They not only perk up in the presence of, but also gesture toward, vocalize, and finally talk about what is unusual. As Roman Jakobson told us many years ago, the very act of speaking is an act of marking the unusual from the usual. (p. 78-79)

Bruner’s text is dense and sometimes difficult to interpret, but this passage made perfect sense to me. We do attend to what is novel. It’s one of the ways we sort through the enormous amount of information we perceive and decide what needs attention.

I didn’t connect this to the Common Core criticisms, though, until just a day or two ago. I read a study about treating bilingualism as a resource in mathematics classrooms that mentioned the very passage from Bruner that I quoted above. In response to the Bruner quote, Dominguez (2011) says, “This may explain why in most mathematics classrooms, students barely talk—they rarely do things with words—as they may be dealing primarily with the usual, that is, repeated exercises of the same kind” (p. 310). This response, like Bruner’s original point, made a lot of sense to me. Familiarity is not always a good thing. It doesn’t always lead to good experiences.

Perhaps this is another way of thinking about the potential benefits of conceptually-based computation methods like counting up. I’ve been focused on explaining why it is not as confusing as some might think. But the other side of that coin is that for some students and in some circumstances, it will be new and confusing. But in the right dosage, that’s ok — even a good thing.

New is what gets people talking. Talking is what gets people learning.

I know it will probably seem like a hollow response to the parents wanting to help kids with their mathematics homework and feeling incapable of doing so. But it at least feels a bit more well-rounded than my previous response insisting that these conceptual methods are not so confusing.

References

Bruner, J. (1990). Entry into Meaning. In Acts of Meaning (pp. 67–97). Cambridge, MA: Harvard University Press.

Common Core State Standards Initiative (CCSSI). (2010). Common Core State Standards for Mathematics. Retrieved from http://www.corestandards.org/Math/ on 1 March 2017.

Domínguez, H. (2011). Using what matters to students in bilingual mathematics problems. Educational Studies in Mathematics, 76(3), 305–328.